If there is one prayer that you should pray/sing every day and every hour, it is the
LORD's prayer (Our FATHER in Heaven prayer)
- Samuel Dominic Chukwuemeka
It is the most powerful prayer.
A pure heart, a clean mind, and a clear conscience is necessary for it.
For in GOD we live, and move, and have our being.
- Acts 17:28
The Joy of a Teacher is the Success of his Students.
- Samuel Chukwuemeka
Measurements and Units

I greet you this day,
First: read the notes.
Second: view the videos.
Third: solve the questions/solved examples.
Fourth: check your solutions with my thoroughly-explained solutions.
Fifth: check your solutions with the calculators. Please follow the directions specified in the
Conversion from Any Unit to Any Unit
Comments, ideas, areas of improvement, questions, and constructive criticisms are welcome. You may contact me.
If you are my student, please do not contact me here. Contact me via the school's system.
Thank you for visiting.
Samuel Dominic Chukwuemeka (Samdom For Peace)
B.Eng., A.A.T, M.Ed., M.S
Objectives
Students will:
(1.) Discuss Dimensional Analysis.
(2.) Discuss Fundamental Quantities.
(3.) Discuss Derived Quantities.
(4.) Discuss the International Metric System (SI) of units for measured quantities.
(5.) Discuss the United States Customary System of units for measured quantities.
(6.) Convert between units for linear measures.
(7.) Convert among units for linear measures.
(8.) Convert between units for quadratic measures/area measures.
(9.) Convert among units for quadratic measures/area measures.
(10.) Convert between units for cubic measures/volume measures.
(11.) Convert among units for cubic measures/volume measures.
(12.) Convert between International Metric System of units and United States Customary System of units.
(13.) Perform arithmetic operations of quantities in the International Metric System.
(14.) Perform arithmetic operations of quantities in the United States Customary System.
(15.) Solve applied problems on Measurements and Units.
(16.) Complete a student project on Measurements and Units.
Skills Measured/Acquired
(1.) Use of prior knowledge
(2.) Critical Thinking
(3.) Interdisciplinary connections/applications
(4.) Technology
(5.) Active participation through direct questioning
(6.) Research
Vocabulary Words
quantity, unit, fundamental quantity, derived quantity, dimensional analysis, dimension, measurement, international system of unit, metric system of unit, international metric system, united states system of unit, customary system of unit, united states customary system, yotta, zetta, exa, peta, tera, giga, mega, kilo, hecto, deka, yocto, zepto, atto, femto, pico, nano, micro, milli, centi, deci,
Introduction
Measurement is the process of defining physical quantities using numbers.
A physical quantity is a physical property of a substance that can be expressed as a number.
Every measurement has a unit.
A unit of measurement is an approved standard for measurement of the same kind of physical quantity.
A system of measurement is a collection of units of measurement and rules relating them to one another.
There are several systems of measurements. However, the three main ones are:
(1.) The International System of Units (Système International) which is also referred to as the Metric System.
(2.) The United States System also referred to as the Customary System.
(3.) The British Imperial System.
For this class, we shall focus on the Metric System and the Customary System.
Physical quantities and units can be classified as:
(1.) Fundamental Quantities and Fundamental Units.
(2.) Derived Quantities and Derived Units.
A fundamental quantity is a basic independent physical quantity that is not defined in terms of another quantity.
A fundamental unit is a basic independent unit that is not defined in terms of another unit.
It is the unit of a fundamental quantity.
| Fundamental Quantity | Fundamental Unit/Symbol | |
|---|---|---|
| (1.) | Length | Meter (m) |
| (2.) | Mass | Kilogram (Kg) |
| (3.) | Time | Second (s) |
| (4.) | Temperature | Kelvin (K) |
| (5.) | Electric Current | Ampere (A) |
| (6.) | Amount of substance | Mole (mol) |
| (7.) | Luminous Intensity | Candela (cd) |
A derived quantity is a physical quantity that is defined in terms of some fundamental quantity.
A derived unit is the unit that is derived from fundamental units.
(Give several examples)
Standardized units are units that have the same meaning for everyone who uses them.
When using standardized units, people can communicate measurements and know that they mean the same thing to both people.
Energy is what makes matter move or heat up.
The units of energy include:
(i.) Joule; the international metric unit for energy
(ii.) Kilowatt-hour; used to measure electrical energy for utility bills.
(iii.) Calorie; used to measure the energy the human body can draw from food.
Power is the rate at which energy is used.
The international metric unit of power is the watt, defined as 1 Watt = 1 Joule per second.
Density is used to describe compactness or crowding.
Common units for density are gram per cubic centimeters (g/cm³), people per square miles (people/mi²), and gigabytes per square inch (GB / inch²).
Concentration is used to describe the amount of one substance that is mixed with another.
Common units for concentration are parts per million (ppm) and milliliters per milligrams (mL/mg).
General Project Requirements
(1.) This is an individual project.
It is not a group project.
Students may work together. However, each student must submit his/her/their own project.
(2.) The items only allowed in the project include containers of:
(a.) Food (includes fruits)
(b.) Drinks (excluding alcoholic drinks)
(c.) Soaps
(d.) Lotion
(e.) Paint
If you are unsure whether any container is permitted, please ask the Professor accordingly.
(3.) The image of the container used must be included.
Please use the Insert → Pictures icon to insert the image directly.
The image should be very clear.

(4.) Please use only the 3 tables given to you.
Any use of any other table will lead to deduction of points.
The only 3 tables are:
| Prefix | Symbol | Multiplication Factor |
|---|---|---|
| yocto | y | $10^{-24}$ |
| zepto | z | $10^{-21}$ |
| atto | a | $10^{-18}$ |
| femto | f | $10^{-15}$ |
| pico | p | $10^{-12}$ |
| nano | n | $10^{-9}$ |
| micro | $\mu$ | $10^{-6}$ |
| milli | m | $10^{-3}$ |
| centi | c | $10^{-2}$ |
| deci | d | $10^{-1}$ |
| deka | da | $10^1$ |
| hecto | h | $10^2$ |
| kilo | K | $10^3$ |
| mega | M | $10^6$ |
| giga | G | $10^9$ |
| tera | T | $10^{12}$ |
| peta | P | $10^{15}$ |
| exa | E | $10^{18}$ |
| zetta | Z | $10^{21}$ |
| yotta | Y | $10^{24}$ |
| Measurement | Customary | Customary | Unit Conversion Factor |
|---|---|---|---|
| Length | inch (in) | foot (ft) | $12\:inches = 1\:ft$ |
| Length | foot (ft) | yard (yd) | $3\:ft = 1\:yd$ |
| Length | yard (yd) | mile (mi) | $1760\:yd = 1\:mi$ |
| Length | foot (ft) | mile (mi) | $5280\:ft = 1\:mi$ |
| Length | rod/pole | yards (yd) | $1\:rod = 5.5\:yd$ |
| Length | furlong | rod | $1\:furlong = 40\;rod$ |
| Length | fathom | feet (ft) | $1\:fathom = 6\;ft$ |
| Length | league/marine | nautical miles | $1\:league = 3\;nautical\;\;miles$ |
| Mass | pound (lb) | ounce (oz) | $1\:lb = 16\:oz$ |
| Mass | short ton (ton) | pound (lb) | $1\:short\:ton = 2000\:lb$ |
| Mass | long ton | pound (lb) | $1\:long\:ton = 2240\:lb$ |
| Mass | stone | pound (lb) | $1\:\:stone = 14\:lb$ |
| Mass | long ton | stone | $1\:long\:ton = 160\:stones$ |
| Area | acre (acre) | square feet ($ft^2$) | $1\:acre = 43560\:ft^2$ |
| Volume | quart (qt) | pint (pt) | $1\:qt = 2\:pt$ |
| Volume | pint (pt) | cup (cup) | $1\:pt = 2\:cups$ |
| Volume | quart (qt) | cup (cup) | $1\:qt = 4\:cups$ |
| Volume | quart (qt) | fluid ounce (fl. oz) | $1\:qt = 32\:fl.\:oz$ |
| Volume | pint (pt) | fluid ounce (fl. oz) | $1\:pt = 16\:fl.\:oz$ |
| Volume | cup (cup) | fluid ounce (fl. oz) | $1\:cup = 8\:fl.\:oz$ |
| Volume | gallon (gal) | quart (qt) | $1\:gal = 4\:qt$ |
| Volume | gallon (gal) | quart (pt) | $1\:gal = 8\:pt$ |
| Volume | gallon (gal) | cup (cup) | $1\:gal = 16\:cups$ |
| Volume | gallon (gal) | fluid ounce (fl. oz) | $1\:gal = 128\:fl.\:oz$ |
| Volume | gallon (gal) | cubic inches ($in^3$) | $1\:gal = 231\:in^3$ |
| Measurement | Metric | Customary | Unit Conversion Factor |
|---|---|---|---|
| Length | meter (m) | foot (ft) | $1\:ft = 0.3048\:m$ |
| Length | nautical miles | kilometer (km) | $1\:nautical\;\;miles = 1.852\;km$ |
| Mass | gram (g) | pound (lb) | $1\:lb = 453.59237\:g$ |
| Mass | metric ton (tonne) | kilogram (kg) | $1\:tonne = 1000\:kg$ |
| Volume |
liter or cubic decimeters (L or $dm^3$) |
gallons (gal) | $1\:L = 0.26417205\:gal$ |
(5.) As a BRCC/VCCS or WNMU student, you have free access to Microsoft Office suite of apps.
(a.) Please download the desktop apps of Microsoft Office on your desktop/laptop (Windows and/or Mac only).
Do not use a chromebook. Do not use a tablet/iPad. Do not use a smartphone.
Do not use the web app/sharepoint access of Microsoft Office.
(Please contact the IT/Tech Support for assistance if you do not know how to download the desktop app.)
In that regard, the project is to be typed using the desktop version/app of Microsoft Office Word only.
(b.) The file name for the Microsoft Office Word project should be saved as: firstName–lastName–project
Use only hyphens between your first name and your last name; and between your last name and the word, project.
No spaces.
(c.) For all English terms (entire project): use Times New Roman; font size of 14; line spacing of 1.5
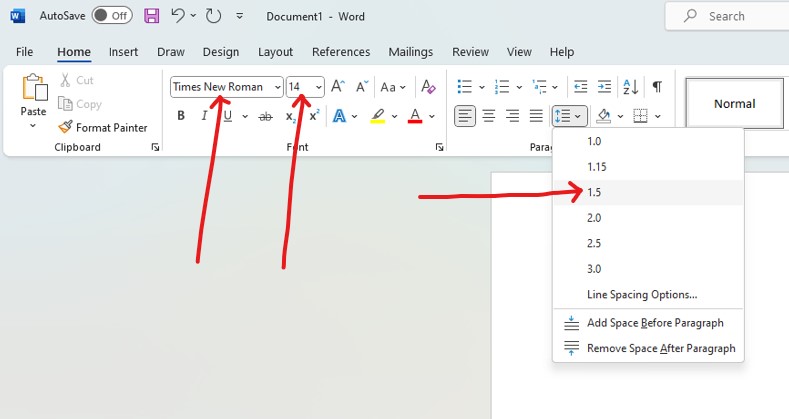
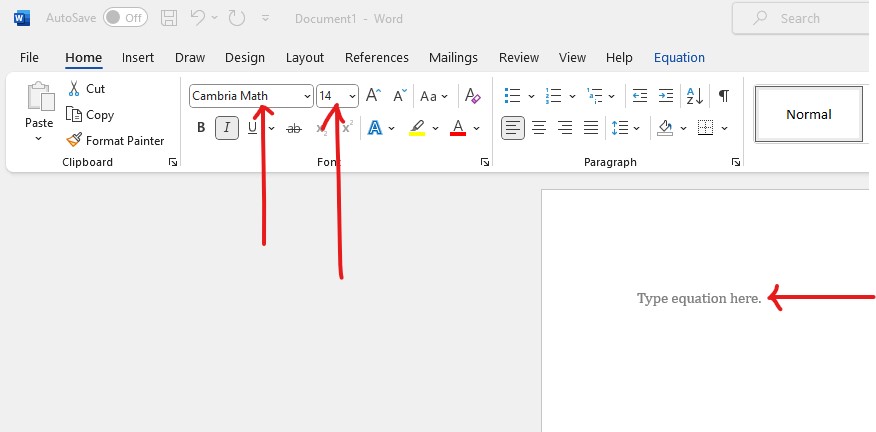
(d.) For all Math terms: symbols, variables, numbers, formulas, expressions, equations and fractions among others,
please use the Math Equation Editor.
(i.) Set the font to Cambria Math; font size of 14; and align accordingly
(ii.) Insert a space after each each equation as applicable. Make a good work that is organized and spacious.

(e.) Include page numbers. You may include at the top of the pages or at the bottom of the pages but not both.

(6.) All work must be shown.
If you use any variables, please define your variables accordingly.
You may use any or a combination of the 3 methods taught/discussed:
(a.) First Method: Unity Fraction Method
(b.) Second Method: Proportional Reasoning Method
(c.) Third Method: Fast Proportional Reasoning Method
If you do not want to use any of these method, you are welcome to use any other pre-approved appropriate method.
(7.) Please ensure your answer matches the converted quantity and unit on the container you used.
Do not approximate intermediate calculations.
If the converted quantity on the container was rounded:
(a.) First, write your answer as is (exact value)
(b.) Second, round accordingly to match the converted quantity on the container. (approximate value)
(c.) Third, specify the type of rounding that was done (how many decimal places, how many significant digits, etc.)
(8.) (a.) Please review the examples I did.
You may not do the same examples that I did.
These are the minimum expectations.
Creativity is always welcome.
NOTE: I did the conversion of one unit to another unit (customary unit to metric unit).
However, please make sure you do two conversions: customary unit to metric unit; and metric unit to customary unit.
(b.) Please review the samples from my previous students also.
You may not submit any of their containers.
(9.) Mr. C (SamDom For Peace) wants you to do this real-world project very well.
Hence, he highly recommends that you submit a draft so he can give you feedback.
(a.) First: (Required): Please submit a clear image of the entire container in the Projects: Containers page in the Canvas course.
The clear image of the entire container should clearly show the units on the container.
I shall review and respond.
(b.) Second: (Highly Recommended): When your container is approved, please submit your draft to me via email or in the Projects: Drafts page in the Canvas
course at least one week prior to the due date.
Please do not submit any draft if the due date is one week or less.
When you submit your draft, I shall review and provide feedback.
When everything is fine (after you make changes as applicable based on my feedback), please submit your work in the
appropriate area in the Canvas course.
Only projects submitted in the Canvas course are graded.
Draft projects are not graded. In other words, projects submitted via email and/or in the Projects: Drafts
page are not graded because they are drafts.
They are only for feedback and should be submitted at least one week prior to the due date.
If it is past one week before the project is due, please do not submit it as a draft to me. Just do it very well
according to the requirements and submit it in the appropriate area (Assignments page: Measurements and Units Project) in the Canvas course.
Submitting drafts is highly recommended. If your professor gives you an opportunity to submit a draft, please use that opportunity.
Submitting drafts is not required. It is highly recommended because I want to give you the opportunity to do your
project very well and make an excellent grade in it.
(10.) All work must be turned in by the final due date to receive credit.
It is highly recommended that you turn in your draft by the first due date. Then, review my feedback and make corrections as necessary before turning in your final
submission by the final due date.
I would not wait till the due dates: the sooner you turn it in, the better.
Examples
NOTE: Unless specified otherwise, please:(1.) Do not approximate intermediate calculations.
(2.) Write the exact value of the answer.
(3.) Write the approximate value of the answer to match the value on the container.
(4.) Specify the type of rounding done to your exact value to match the value on the container.
| Name: | (Registered name as is in the Canvas course) |
| Instructor: | Samuel Chukwuemeka |
| Objective: | To convert a measurement from a unit to another unit. |
| Measurement: | Mass |
| 1st: Given Unit: | Customary unit (Ounce) |
| To Convert to: | Metric unit (Gram) |
| 2nd: Given Unit: | Metric unit (Gram) |
| To Convert to: | Customary unit (Ounce) |
| Container Used: | Soap (Please see the bottom left corner.) |

|
|
Convert $4.25\:oz$ to $g$
From Given Tables:
$
1\:lb = 16\:oz \\[3ex]
1\:lb = 453.59237\:g \\[3ex]
1\:lb = 1\:lb \\[3ex]
\implies 16\:oz = 453.59237\:g \\[3ex]
Let\:\:p = mass\:\:of\:\:4.25\:oz\:\:in\:\:g \\[3ex]
\underline{Second\:\:Method:\:\:Proportional\:\:Reasoning\:\:Method} \\[3ex]
$
| $oz$ | $g$ |
|---|---|
| $16$ | $453.59237$ |
| $4.25$ | $p$ |
$ \dfrac{p}{4.25} = \dfrac{453.59237}{16} \\[5ex] Multiply\:\:both\:\:sides\:\:by\:\:4.25 \\[3ex] 4.25 * \dfrac{p}{4.25} = 4.25 * \dfrac{453.59237}{16} \\[5ex] p = \dfrac{4.25 * 453.59237}{16} \\[5ex] p = \dfrac{1927.76757}{16} \\[5ex] p = 120.485473 \\[3ex] p \approx 120\:g...rounded\:\:to\:\:the\:\:nearest\:\:whole\:\:number \\[3ex] \therefore 4.25\:oz \approx 120\:g \\[3ex] $ This confirms the quantity in $g$ (in parenthesis) in the soap container.
| Name: | (Registered name as is in the Canvas course) |
| Instructor: | Samuel Chukwuemeka |
| Objective: | To convert a measurement from a unit to another unit. |
| Measurement: | Volume |
| 1st: Given Unit: | Customary unit (Fluid Ounce) |
| To Convert to: | Metric unit (Milliliters) |
| 2nd: Given Unit: | Metric unit (Milliliters) |
| To Convert to: | Customary unit (Fluid Ounce) |
| Container Used: | Water (Please see the top center corner.) |

|
|
Convert $16.9\:fl\:\:oz$ to $mL$
From Given Tables:
$
1\:L = 0.26417205\:gal \\[3ex]
1\:gal = 4\:qt \\[3ex]
1\:qt = 4\:cups \\[3ex]
1\:cup = 8\:fl.\:oz \\[3ex]
$
Based on what we were given:
Let us first convert it to liters ($L$)
Then, we will convert from liters ($L$) to milliliters ($mL$)
$
\underline{First\:\:Method:\:\:Unity\:\:Fraction\:\:Method} \\[3ex]
16.9\:fl\:oz\:\:to\:\:L \\[3ex]
Set\:\:it\:\:up\:\:and\:\:check\:\:to\:\:make\:\:sure\:\:it\:\:is\:\:correct \\[3ex]
16.9\:fl\:oz * \dfrac{.....L}{.....gal} * \dfrac{.....gal}{.....qt} * \dfrac{.....qt}{.....cup} * \dfrac{.....cup}{.....fl.\:oz} \\[5ex]
16.9\:fl\:oz * \dfrac{1\:L}{0.26417205\:gal} * \dfrac{1\:gal}{4\:qt} * \dfrac{1\:qt}{4\:cup} * \dfrac{1\:cup}{8\:fl.\:oz} \\[5ex]
= \dfrac{16.9 * 1 * 1 * 1 * 1}{0.26417205 * 4 * 4 * 8} \\[5ex]
= \dfrac{16.9}{33.8140224} \\[5ex]
= 0.4997926541\:L \\[3ex]
Convert\:\:0.4997926541\:L\:\:to\:\:mL \\[3ex]
\underline{First\:\:Method:\:\:Unity\:\:Fraction\:\:Method} \\[3ex]
Set\:\:it\:\:up\:\:and\:\:check\:\:to\:\:make\:\:sure\:\:it\:\:is\:\:correct \\[3ex]
0.4997926541\:L * \dfrac{.....mL}{.....L} \\[5ex]
= 0.4997926541\:L * \dfrac{1\:mL}{10^{-3}\:L} \\[5ex]
= 0.4997926541\:L * \dfrac{1\:mL}{0.001\:L} \\[5ex]
= 499.7926541\:mL \\[3ex]
499.7926541\:mL \approx 500\:mL \\[3ex]
$
This confirms the quantity in $mL$ in the water container.
Student: Sir, you could have used the direct conversion from gallons to cups...
and bypass quarts
$
16.9\:fl\:oz * \dfrac{.....L}{.....gal} * \dfrac{.....gal}{.....cup} * \dfrac{.....cup}{.....fl.\:oz} \\[5ex]
16.9\:fl\:oz * \dfrac{1\:L}{0.26417205\:gal} * \dfrac{1\:gal}{16\:cups} * \dfrac{1\:cup}{8\:fl.\:oz} \\[5ex]
$
Teacher: That is right!
You are correct.
But, what if you were given a table that does not have that direct conversion?
Student: Then, I would use what I was given.
But, in this case; we were given that direct conversion.
| Name: | (Registered name as is in the Canvas course) |
| Instructor: | Samuel Chukwuemeka |
| Objective: | To convert a measurement from a unit to another unit. |
| Measurement: | Lengths (Width by Length) |
| 1st: Given Unit: | Customary unit (in by in) |
| To Convert to: | Metric unit (cm by cm) |
| 2nd: Given Unit: | Metric unit (cm by cm) |
| To Convert to: | Customary unit (in by in) |
| Container Used: | Hand Wipes (Please see the bottom center corner.) |

|
|
Convert $5.7\:in\:\:by\:\:7.5\:in$ to $cm\:\:by\:\:cm$
$
Width = 5.7\:in \\[3ex]
Length = 7.5\:in \\[3ex]
$
From Given Tables:
$
1\:ft = 0.3048\:m \\[3ex]
12\:inches = 1\:ft \\[3ex]
1\:ft = 12\:inches \\[3ex]
1\:ft = 1\:ft \\[3ex]
\implies 0.3048\:m = 12\:inches \\[3ex]
$
We shall use the First Method to convert the width.
We shall use the Second Method to convert the length.
Use any method(s) you prefer.
$
\underline{First\:\:Method:\:\:Unity\:\:Fraction\:\:Method} \\[3ex]
Convert\:\:the\:\:Width \\[3ex]
5.7\:in \:\:to\:\: cm \\[3ex]
Set\:\:it\:\:up\:\:and\:\:check\:\:to\:\:make\:\:sure\:\:it\:\:is\:\:correct \\[3ex]
5.7\:in * \dfrac{.....m}{.....in} * \dfrac{.....cm}{.....m} \\[5ex]
5.7\:in * \dfrac{0.3048\:m}{12\:in} * \dfrac{1\:cm}{10^{-2}\:m} \\[5ex]
= 5.7\:in * \dfrac{0.3048\:m}{12\:in} * \dfrac{1\:cm}{0.01\:m} \\[5ex]
= \dfrac{5.7 * 0.3048 * 1}{12 * 0.01} \\[5ex]
= \dfrac{1.73736}{0.12} \\[5ex]
= 14.478\:cm \approx 14.5\:cm \\[3ex]
\underline{Second\:\:Method:\:\:Proportional\:\:Reasoning\:\:Method} \\[3ex]
Convert\:\:the\:\:Length \\[3ex]
Let\:\:p = length\:\:of\:\:7.5\:in\:\:in\:\:m \\[3ex]
Let\:\:c = length\:\:of\:\:p\:m\:\:in\:\:cm \\[3ex]
$
Based on what we were given:
We need to first convert to meters ($m$)
Then, we will convert from meters ($m$) to centimeters ($cm$)
From Given Tables:
$
1\:ft = 0.3048\:m \\[3ex]
12\:inches = 1\:ft \\[3ex]
1\:ft = 12\:inches \\[3ex]
1\:ft = 1\:ft \\[3ex]
\implies 0.3048\:m = 12\:inches \\[3ex]
1\:cm = 10^{-2}\:m \\[3ex]
1\:cm = 0.01\:m
$
| $in$ | $m$ |
|---|---|
| $12$ | $0.3048$ |
| $7.5$ | $p$ |
$ \dfrac{p}{7.5} = \dfrac{0.3048}{12} \\[5ex] Multiply\:\:both\:\:sides\:\:by\:\: 7.5 \\[3ex] 7.5 * \dfrac{p}{7.5} = 7.5 * \dfrac{0.3048}{12} \\[5ex] p = \dfrac{7.5(0.3048)}{12} \\[5ex] p = \dfrac{2.286}{12} \\[5ex] p = 0.1905\:m \\[3ex] $
| $m$ | $cm$ |
|---|---|
| $0.01$ | $1$ |
| $0.1905$ | $c$ |
$ \dfrac{c}{1} = \dfrac{0.1905}{0.01} \\[5ex] c = 19.05\:cm \approx 19.1\:cm $
The results confirm the quantities in cm in the hand wipe container.
Student Project Samples
First Sample: Daneker: Cheez It: Baked Snack Crackers BoxSecond Sample: Rachel: Bubble Bath Soap Bottle
Third Sample: Groff: Garlic Powder Container
Fourth Sample: Stephanie: Tapatio Hot Sauce
Fifth Sample: Elizabeth: Pillsbury White Frosting
Sixth Sample: Taylor: Bubble Gel Cleanser
Seventh Sample: Mikalah: Dr. Pepper bottle
The teacher should guide each student to the successful completion of the project.
Let students know you are willing to help.
References
Chukwuemeka, S.D (2016, April 30). Samuel Chukwuemeka Tutorials - Math, Science, and Technology.
Retrieved from https://www.samuelchukwuemeka.com
Bennett, J. O., & Briggs, W. L. (2019).
Using and Understanding Mathematics: A Quantitative Reasoning Approach. Pearson.
CrackACT. (n.d.). Retrieved from http://www.crackact.com/act-downloads/
CMAT Question Papers CMAT Previous Year Question Bank - Careerindia. (n.d.). https://www.careerindia.Com. Retrieved May 30, 2019,
from https://www.careerindia.com/entrance-exam/cmat-question-papers-e23.html
CSEC Math Tutor. (n.d). Retrieved from https://www.csecmathtutor.com/past-papers.html
Essentials of the SI: Base & derived units. (2019). Nist.gov. https://physics.nist.gov/cuu/Units/units.html
MySchoolGist - Free West African Senior School Certificate Examination (WASSCE) Past Questions. (n.d). Retrieved from https://www.myschoolgist.com/ng/free-waec-past-questions-and-answers/
National Institute of Standards and Technology, U.S Department of Commerce - The International System of Units (SI). (n.d). Retrieved from https://www.nist.gov/sites/default/files/documents/2016/12/07/sp330.pdf
Free Jamb Past Questions And Answer For All Subject 2020. (2019, January 31). Vastlearners. https://www.vastlearners.com/free-jamb-past-questions/
Mathematics. (n.d.). waeconline.org.ng. Retrieved May 30, 2020, from https://waeconline.org.ng/e-learning/Mathematics/mathsmain.html
NSC Examinations. (n.d.). www.education.gov.za. https://www.education.gov.za/Curriculum/NationalSeniorCertificate(NSC)Examinations.aspx
51 Real SAT PDFs and List of 89 Real ACTs (Free) : McElroy Tutoring. (n.d.).
Mcelroytutoring.com. Retrieved December 12, 2022,
from https://mcelroytutoring.com/lower.php?url=44-official-sat-pdfs-and-82-official-act-pdf-practice-tests-free